


|
Frank Werner, Steffen Frik
Adam Opel AG
Josef Schulze
The requirements for racing car aerodynamics are far more extensive and demanding than those for passenger cars. Since many of the relevant aerodynamic features cannot be measured easily, if at all, Computational Fluid Dynamics (CFD) provides a detailed insight into the flow phenomena and helps in understanding the underlying physics.
This paper summarizes some aspects of the aerodynamic optimization process for the Opel Calibra ITC racing car, starting from the production car design and including exterior and interior aerodynamic computations, together with wind tunnel experiments.
The design regulations for the Class 1 Racing Car Championship ITC, as for its predecessor DTM, have become increasingly more liberal. It has proved necessary to add highly sophisticated aerodynamic features, in order to improve the aerodynamics of the racing cars and hence achieve competitiveness. This has resulted in greater technical differences between racing cars and their corresponding production vehicles.
Fig.1 explains the enormous significance of drag optimization for racing cars. Due to the high speeds, a small increase in drag leads to a substantial rise in the required engine power needed to overcome this drag. For example, the Calibra racing car has a drag coefficient (CD) of about 0.36 for a particular setup, whereas the value for the equivalent Calibra production vehicle is only 0.26, which is the lowest for all production passenger cars. The higher drag coefficient of the Calibra racing car is mainly caused by the rear wing, needed to provide the desired downward force. All values in Fig.1 concerning the required additional engine power are given with respect to this particular setup.
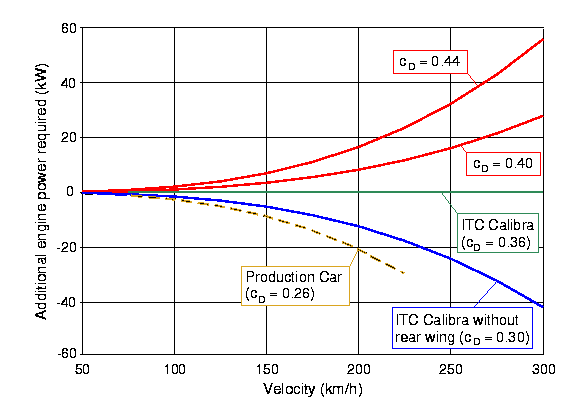 Fig. 1 Engine power required for different drag coefficients |
The other drag coefficients mentioned in Fig.1 describe the range of other setups and the estimated values of some competitor cars. It is obvious that these differences have a decisive effect on possible accelerations and speeds, and hence may decide the race.
Since Class 1 racing cars are so powerful (approx. 380 kW), many other factors must also be carefully considered. For example, the required downward forces and balancing must be provided without significantly impairing the drag coefficient. In addition, the cooling systems for the engine, brakes, and electronic devices and the efficiency of the engine intake system are of vital importance.
To fulfill these tasks, the following aerodynamic features were developed and applied to the Opel ITC racing car Calibra (Fig.2):
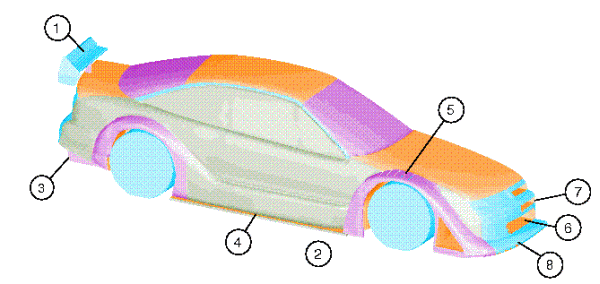 Fig. 2 Aerodynamic features of the OPEL Calibra ITC racing car |
rear wing with gurney (1)
underbody almost completely covered (2)
diffuser channels separated by strakes (3)
wooden bars at each side of the vehicle to reduce the underbody flow leakage (4)
wheel-house ventilation (5)
variable device using a set of flaps to shut the inlet of the engine cooling duct (6)
brake cooling duct (7)
front splitter (8)

|
The development of racing cars is characterized by extremely short design cycles. Hence, to achieve all the required objectives, there must be a very close interaction between experimental and computational activities. In addition, each engineering discipline must focus on its own particular strengths in order to maximize effectiveness.
In the past, aerodynamic development was mainly performed in wind tunnels, ignoring the relative movement between the vehicle and the ground. However, our preliminary wind tunnel tests, performed with rotating as well as non-rotating wheels, showed that the relative movement of the vehicle with respect to the ground has a significant effect on the underbody flow (Fig.3). All values in Fig.3 are denoted with respect to the drag coefficient for the baseline racing car with fixed wheels and ground.
 Fig. 3. Impact of different wind tunnel setups on drag coefficient |
The importance of more sophisticated tests can be illustrated as follows: measurements for cars with non-rotating wheels indicated that only one modification - completely covering the underbody - leads to an improved drag coefficient, whereas all other options increase the drag. However, in contrast to these results, the realistic setup, which included rotating wheels and movement relative to the ground, showed improvements for all cases. A general rule is that a test gives higher drag coefficients when performed with rotating wheels than with non-rotating wheels. Considering the very low ground clearance of a racing car (approx. 30 mm) this effect could be predicted. Consequently, all wind tunnel tests and computational models, especially those used to simulate underbody flow phenomena, must include rotating wheels and movement relative to the ground.
The following paragraphs describe some of the aerodynamic features mentioned above, in more detail:
 | |
 |
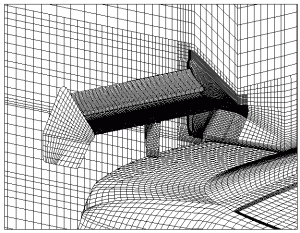 |
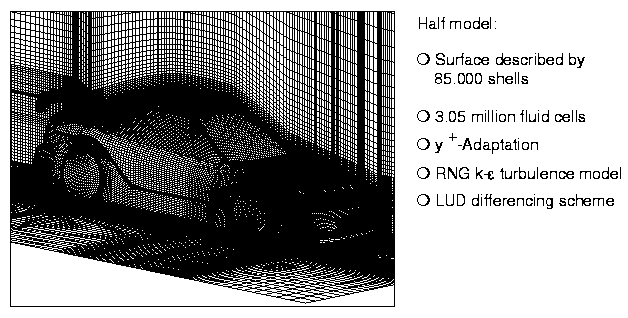
| Fig. 4. Computational Model | |
In addition to numerous wind tunnel tests, the development of most of the aerodynamic features was supported extensively by Computational Fluid Dynamics (CFD). A three-dimensional wind tunnel model was created, containing 3.6 million fluid cells. The fine detail of this computational mesh can be seen in Fig.4, which displays the surface grid for the vehicle. The turbulent flow is calculated with the CFD code STAR-CD [3], solving Reynolds-averaged Navier-Stokes equations with a RNG k-e turbulence model [4]. The computational model included all relevant aerodynamic features mentioned above (Fig.2), and assumed the racing car to be symmetric. All main interior ducts (engine cooling, brake cooling, airbox intake) had to be modelled, as the interaction of the external and internal flows was part of the investigation. Rotating wheels and movement relative to the ground were included, in order to simulate realistic road conditions.
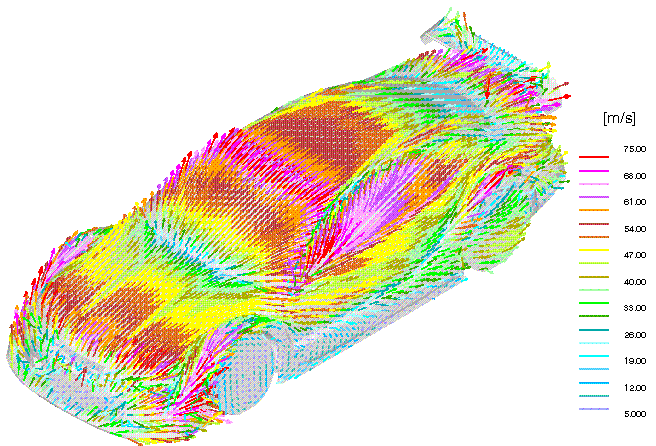 Fig. 5. Calculated Flow velocities near surface (rotating wheels and moving ground) |
The calculated flow velocities shown in Fig.5 and the cp-distribution near the surface of the car, see Fig.6, give an overall view of the surface flow characteristics of the ITC Calibra racing car.
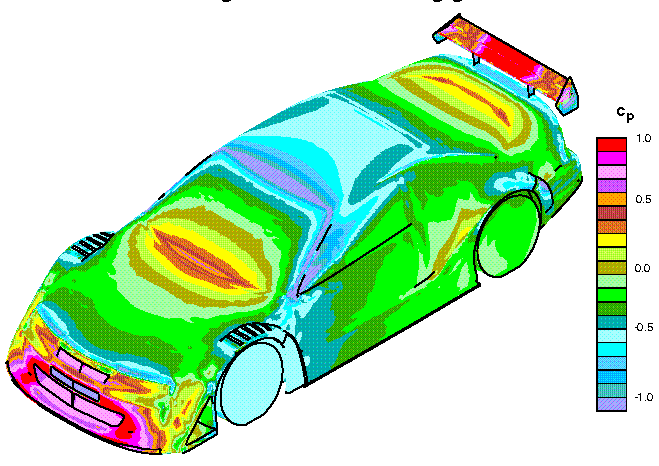 Fig. 6. Calculated pressure distribution on surface (rotating wheels and moving ground) |
Due to the huge size of the computational grid, the aerodynamics simulation of the complete racing car took too much modelling and computing time for setup optimization or sensitivity studies to be performed. Consequently, the aim of the CFD work was to predict trends and to achieve a better understanding of qualitative flow characteristics, rather than to calculate values such as drag or lift coefficients. This approach seems to be the only feasible one as current computer codes are not able to predict drag and lift with the required accuracy [5]. Certain tasks such as setup optimizations, which are characterized by very small design changes e.g. an inclination or offset of the rear wing, can be performed much more quickly by means of experimental devices than by computational analyses.
Some simulations were performed using simplified submodels, which included all relevant aerodynamic features, in order to save time.

|
ENGINE COOLING DUCTS - The main tasks were to achieve a uniform flow through the radiator and to minimize the interaction of the external flow and the flow leaving the duct. Here, a simplified model of the front body was used to enable easier geometry modifications and faster turnaround. The vehicle was assumed to be symmetric, so that a half model of the front body, from the front splitter to the B-pillar, was employed. The underbody flow and the wheel rotation were not included.
The baseline geometry led to a relatively strong interaction between the external flow and the flow exiting the cooling duct, which generated an extended flow separation near the front wheel (Fig.7a). This large recirculation zone widened the vehicle's "aerodynamic effective cross-section", so that the drag increases.
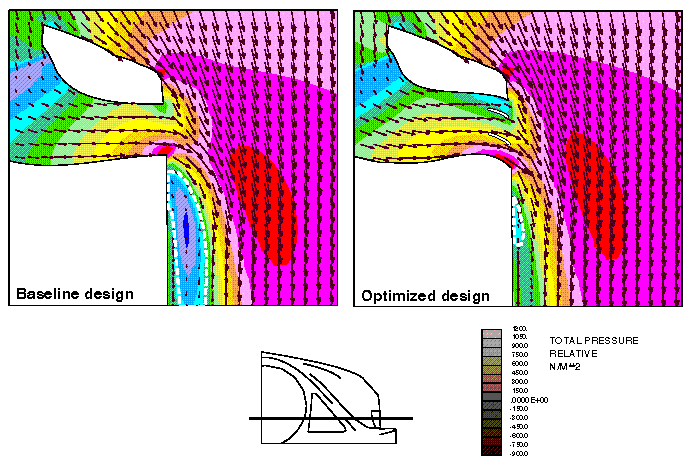 Fig. 7. Horizontal section through cooling duct (Simplified Model) |
Several duct shapes were analyzed in order to minimize this effect. Due to package restrictions for the wheel house and front fender regions, nearly all the modifications had to be carried out within the envelope of the baseline duct. An additional constraint was that the air flow through the radiator must remain uniform. All these requirements made the use of CFD essential for a systematic optimization strategy.
The interaction among the two flow streams was considerably reduced (Fig.7b) by shape modifications between the radiator and the outlet and by the introduction of vanes. These vanes were inserted at the duct exit in order to deflect the air flow leaving the cooling duct, so that it became nearly tangential to the external flow. Thus, the wake next to the front wheels almost disappeared.
A device to shut the cooling duct helped to completely avoid the interaction of the two flows. This device consisted of several flaps, activated automatically by vehicle speed and coolant temperature. The orientation of the flaps at the fully open position was defined by the calculated flow velocities at the inlet plane of the duct. Thus, the effect of the flaps on the flow for the fully open position could be minimized. With this device, when the flaps are closed the drag coefficient can be reduced by up to approximately 0.02.
FRONT END AERODYNAMICS - Various front end configurations were simulated, in order to determine how the external flow was affected by the internal flows through the airbox, brake and engine cooling systems. Different internal flows were considered, starting with a completely closed front, which of course is not feasible. It was assumed that the vehicle moved at 250 km/h and that the engine was running at maximum rpm (approx. 12.000 rpm). Fig.8 displays the normalized pressure distribution in the symmetry plane. Clearly, the internal flows led to considerable changes in the front end pressure distribution. In particular, the low pressure region at the front of the hood (case 1) almost disappeared when the internal ducts were open (cases 3 and 4). This effect is more important for racing cars than for ordinary passenger cars, as racing car engines operate at higher rpms for a given vehicle speed so that the ratio of the air flow through the airbox to the external flow is much higher.
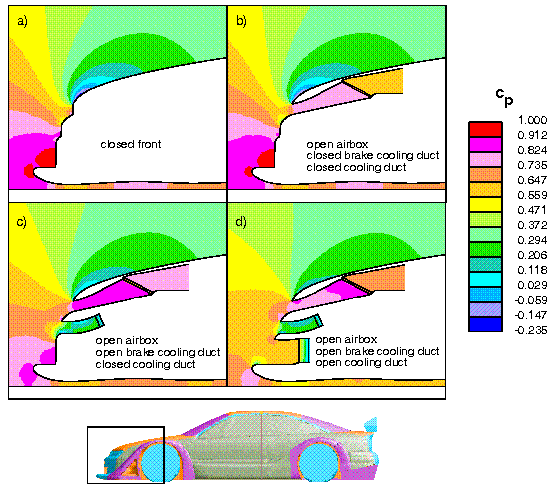 Fig. 8. Pressure distribution for different front end configurations |
These results demonstrate that the internal flows must be taken into account for an accurate prediction of the front end flow.

|
REAR WING - Since the Calibra is shaped like a coupe, the rear wing configuration was of major concern. Systematic experimental and computational studies were performed with different multi-component airfoil configurations and gurney lengths, in order to achieve both low drag and well balanced maximum downward forces on the axles.
These studies revealed an important rear design consideration: Fig.9 shows the computed air flow velocity vectors near the rear wing for the early Calibra styling body (production car) quantifying the flow angle close to the leading edge of the rear wing. It was found that the inflow velocity vector changed its orientation from middle to side body section. The rear spoiler in the current vehicle was redesigned to perform in this way and was then measured in the wind tunnel. The new design led to an 8% increase in downward force, with the same drag force as a conventional design with constant wing angles. Fig.10 displays the calculated flow field and pressure distribution for this optimized multi-component airfoil. Overall, the experimental and computational optimization indicated that coupé-styled racing cars need to be treated differently to notchback cars.
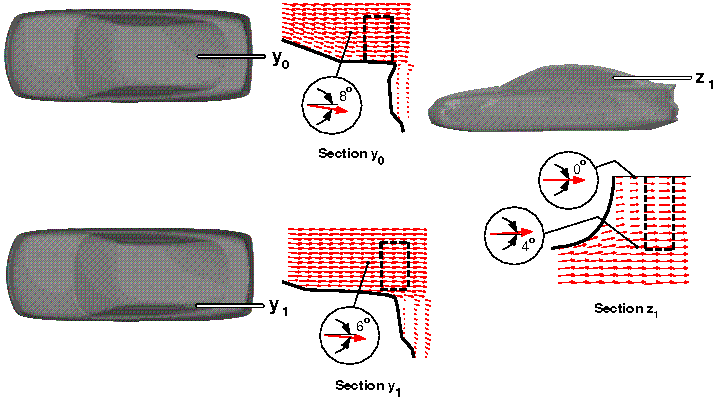 Fig. 9. Inflow vector near leading edge of rear wing (Styling model of production Calibra) |
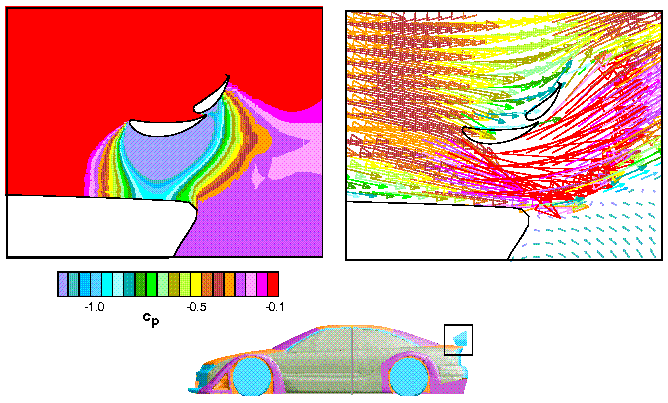 Fig. 10. Flow field and pressure distribution at rear wing |
DIFFUSER - The diffuser is an important means of increasing the downward force at the rear axle. The optimum diffuser angle for lift and drag was determined by an extensive series of wind tunnel tests. These tests were performed in the wind tunnel in Emmen, Switzerland, which has facilities to simulate rotating wheels and movement relative to the ground.
The results of the three-dimensional simulation of the complete racing car showed that the flow around the rear wheels disturbed the flow at the diffuser intake, so that flow separations occured inside the diffuser channels (Fig.11a).
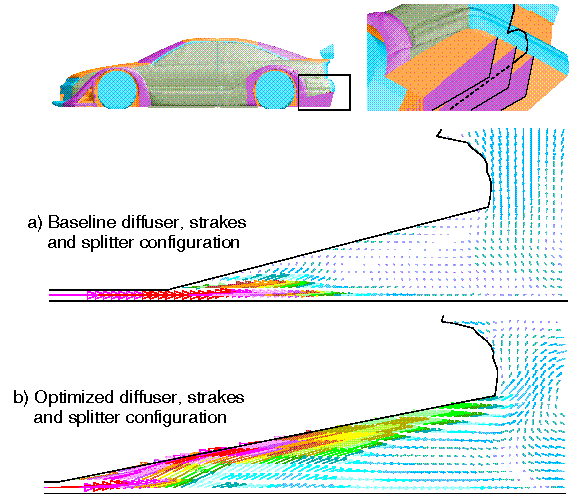 Fig. 11. Calculated diffuser flow (Baseline/Optimised) |
In order to improve the diffuser performance, firstly the diffuser angle was optimized. Additionally, strakes were used to subdivide this region into several separate channels, reducing leakage of the underbody flow. Furthermore the front splitter and the position of the strakes were modified to increase and direct the underbody flow and hence reduce the influence of the flow around the wheels. Due to these design modifications, the flow was then completely attached to the upper side of the diffuser (Fig.11b), giving a low pressure level at the rear underbody. The higher underbody flow rate additionally decreased the static pressure and thereby led to a higher downward force.
Aerodynamics optimization is of vital importance in achieving competitiveness for racing cars, because of their very high performance levels. The extremely short design cycle for such a vehicle requires very close cooperation between experimental and computational development work.
Experimental data and computational results together show that the simulation of racing car aerodynamics must include the modelling of all aerodynamic car features. The optimization strategy for the Opel Calibra racing car involved the following:
Internal flows (airbox, engine and brake cooling duct) must be taken into account to simulate the correct front end body flow. The re-entering of these flows into the external flow is of major importance, because they can have a major effect on external flow characteristics.
The very low ground clearance of racing cars means that rotating wheels and movement relative to the ground must be modelled in order to accurately predict the underbody flow. Measured data show that neglecting the relative movement of the vehicle and the wheels with respect to the ground may sometimes lead to completely wrong results when determining the influence of geometry modifications on the lift and drag coefficients. This means that a model with non- rotating wheels and fixed with respect to the ground cannot even predict trends reliably.
To achieve the required results in time, some analyses had to be performed using simplified computational models. However, even these models contained all significant local aerodynamic features. This aerodynamic optimization, characterized by the simultaneous application of experimental and computational tools, together with the close cooperation of all engineers involved, was part of the success of the Opel Calibra Class 1 racing car, winning the ITC Championship in 1996.
The authors thank F. Ross, adapco, who performed some of the flow calculations.
H. Emmelmann, H. Berneburg, J. Schulze, The Aerodynamic Development of the Opel Calibra, SAE Paper 900317
F. Indra, Welche Vorteile bringt der Motorsport fur die Serienentwicklung, Automobil Revue 16, 1994
Computational Dynamics Ltd., STAR-CD Manual Version 3.0, 1997
V. Yakhot, S.A. Orszag, Renormalization group analysis of turbulence. J. Scientific Computing, 1:1-51, 1992
M. Ramnefors et al., Accuracy of Drag Predictions on Cars Using CFD - Effect of Grid Refinement and Turbulence Models, SAE Paper 960681